Monogenic field

In mathematics, a monogenic field is an algebraic number field K for which there exists an element a such that the ring of integers O_K is the polynomial ring Z[a]. The powers of such an element a constitute a power integral basis.

In a monogenic field K, the field discriminant of K is equal to the discriminant of the minimal polynomial of α.

Examples

Examples of monogenic fields include:

Quadratic fields:

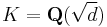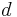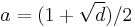if $K = \mathbf{Q}(\sqrt d)$ with $d$ a square-free integer, then $O_K = \mathbf{Z}[a]$ where $a = (1%2B\sqrt d)/2$ if d≡1 (mod 4) and 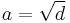$a = \sqrt d$ if d ≡ 2 or 3 (mod 4).

Cyclotomic fields:

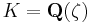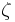if $K = \mathbf{Q}(\zeta)$ with $\zeta$ a root of unity, then $O_K = \mathbf{Z}[\zeta].$

Not all number fields are monogenic; Richard Dedekind gave the example of the cubic field generated by a root of the polynomial 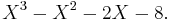$X^3 - X^2 - 2X - 8.$

References

Narkiewicz, Władysław (2004). Elementary and Analytic Theory of Algebraic Numbers. Springer-Verlag. pp. 64. ISBN 3540219021.
Gaál, István (2002). Diophantine Equations and Power Integral Bases. Birkhäuser Verlag. ISBN 978-0-8176-4271-6.